Assumptions When Finding Area Examples

Let R be the region bounded by the graph of y = x² and the line y = 4. Write an integral expression for the area of R, using

(a) vertical slices

(b) horizontal slices

First we have to make sure we know what R is. Draw the graph y = x² and the line y = 4:

Then shade in the region they surround:

We could slice this area either way. Sometimes it'd easier to evaluate the integral if we slice it one way instead of the other. We'll see what we get from slicing each way here.

(a) If we slice vertically, the variable of integration is x. The variable x tells the distance of the slice from the y-axis:

The height of a slice is 4 – x²:

This means the area of a slice is

(4 – x²) Δ x.

Since we're integrating with respect to x, the limits of integration are whatever the least and greatest reasonable values are for x.

The line y = 4 intersects the graph y = x² when x = ± 2.

So the limits of integration are from -2 to 2, and the area of R is . There's another option that's even easier. Since the region R consists of two identical pieces, we can integrate from 0 to 2 and multiply the result by 2. Another expression for the area of R is . This area is simple to integrate, but we won't do it here.

(b) If we slice horizontally, then y is the variable of integration. The variable y tells us the "height" of the slice above the x-axis.

The slice at height y has right endpoint (x,y) on the graph of y = x², so we could also write the coordinates of this endpoint as . The width of the slice is twice the x-coordinate, or :

This means the area of the slice is . The slice at the bottom of R is at "height" y = 0, and the slice at the top is at "height" y = 4. So the limits of integration are from 0 to 4, and the area of R is . This area is more difficult to integrate than the vertical slices. We could do it here, but we should be glad we don't have to. We dodged a bullet.

Sometimes a region is shaped so oddly that we have to turn our heads and rub our eyes just to make sure we aren't hallucinating. Yes, we will still have to integrate it. We just have to be smart about the way we slice it, because it affects whether or not we can express its area with just one integral. We might have to split the region into pieces and find the area of each piece separately. In these cases, an "integral expression" may consist of more than one integral.

Let R be the region between the graphs , y = x², and the line x = 4. Write an integral expression for the area of R using horizontal slices.

The region R looks like this: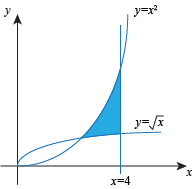

Since the problem says to use horizontal slices, life will be a little bit difficult. We could hit our heads on the wall and hope it works itself out, but it won't. We might as well dive in head first.

A slice at the bottom of the region has its left endpoint on the line y = x² and its right endpoint on the line .

Meanwhile, a slice at the top of the region has its right endpoint on the line x = 4.

Don't throw your pencil at a wall. It's easier to handle than you may think. We have to split the region into two pieces: one piece where the horizontal slices go from the graph y = x² to the graph , and one piece where the horizontal slices go from the graph y = x² to the line x = 4. Let's look at the lower piece first, and start by figuring out the limits of integration.

We know that y = x² and intersect at (1,1), so y = 1 will be our lower limit of integration. The upper limit of integration is where the graph  intersects the line x = 4, which is at (4,2). The upper limit of integration is y = 2.

The slice at position y goes from a point on the graph of y = x² with coordinates  to a point on the graph of  with coordinates

(x,y) = (y², y).

The length of this slice is , so its area is

Writing the integral with the limits of integration we figured out earlier, the area of this piece of the region is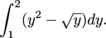

For the upper piece of R, the region goes from y = 2 to y = 4² = 16.

A slice extends from the graph of y = x² to the line x = 4.

The width of a slice is , so the area is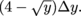

This means the area of this piece of the region is

Now that we have two areas, we just need to add them together to get the total area of the region R: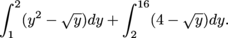

That was quite a bit of work. It would have been faster to use vertical slices, but the evil Shmoop question writing demon felt a little torture was in order. Despite our growling stomachs from all the talk about fish sticks, we solved it.