ShmoopTube
Where Monty Python meets your 10th grade teacher.
Search Thousands of Shmoop Videos
Physics Videos 34 videos
Isaac Newton. Who was he? Why do we need to know about him? In a physics course, no less? Well, he's only the most famous physicist in history, and...
What are the basics of trigonometry? And why are we learning about this in a physics course? Both good questions. In this video, you'll learn about...
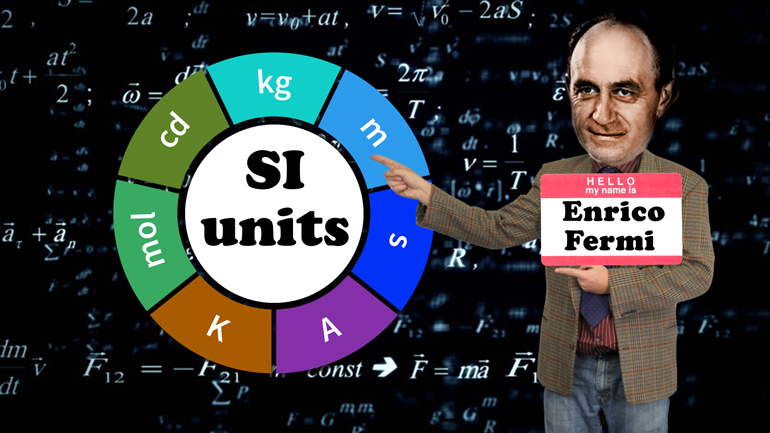
It's time to make our liters and meters work together. Enough of the bickering, right? In this video, we'll do some unit analysis, covering SI Unit...
Physics: Unit Analysis and Graphical Data Analysis 36 Views
Share It!
Description:
It's time to make our liters and meters work together. Enough of the bickering, right? In this video, we'll do some unit analysis, covering SI Units, isolating variables, setting up graphs, slopes, and curves. And there will be algebra. Oh, yes - there will be algebra.
Transcript
- 00:03
unit analysis and geographical data analysis who spilled all these letters
- 00:07
in my math unit analysis make your leaders and meters work together...[mumbling]
- 00:56
Alright let's get started hope you're in the mood for some algebra [Ring beaming colors]
- 01:00
remember the Pythagorean theorem a squared plus B squared equals C squared
- 01:06
looks pretty algebraic to us and when we're dealing with formulas in physics
Full Transcript
- 01:11
we've got to be able to solve for the different variables we'll be using
- 01:15
so we'll need to isolate those variables and make sure we're using the right [Variables in a prison cell]
- 01:19
units at the same time and using the right units it is important we're going
- 01:25
to be dealing with more than just meters and seconds we'll be looking at things
- 01:29
like kilograms times meters per second squared and sometimes we're going to be
- 01:34
dealing with equations where we just want to see how the unit's involved
- 01:37
relate with each other without plugging in any specific numbers which is called
- 01:41
unit analysis we'll put unit analysis to work when we need to solve for a
- 01:47
particular variable and once we've isolated that variable that's when we [Hand picks up E variable]
- 01:51
can plug in any numbers that we have so how do we isolate a variable well the
- 01:57
laws of algebra say that anything we do to one side of an equation we do to the
- 02:01
other side as well algebra is all about equality so if [Man and woman sitting on a seesaw]
- 02:06
we've got an equation that says A plus B C over D and we want to solve for A then
- 02:14
we have to subtract B from both sides meaning A equals C over D minus B and
- 02:21
when we're doing algebra or any math really we can't forget the order of [PEMDAS appears with corresponding symbols]
- 02:26
operations and we can't forget that when we've got PEMDAS lodged into our brain
- 02:31
yep another nonsense math word PEMDAS tells us what to do first when we're
- 02:37
dealing with a complicated piece of math it's also called the order of operations
- 02:43
so we solve anything in parentheses first then we handle the exponents after
- 02:49
that comes multiplication and division and really those two have equal standing
- 02:54
in the land of PEMDAS so if you have a multiplication and division in a problem
- 02:58
just solve them going left to right and bringing up the rear are addition and
- 03:04
subtraction those two are also equivalent so do the left to right thing
- 03:08
with them too okay okay enough talk let's see how we apply this stuff to a [Woman discussing unit analysis]
- 03:14
physics problem so you know how there's the Sun and then there's the earth where
- 03:21
you know we're all just chillin and you know how they're really far away from [Earth and Sun in space and boy appears scratching head]
- 03:26
each other let's play around with stuff to find out just how far apart they are
- 03:30
and as you might expect we'll start with gravity the force of
- 03:34
gravity between two masses like the Sun and the earth is given by the equation F
- 03:39
sub G for the force of gravity equals G which is the gravitational constant
- 03:44
times the mass of the first body M sub one times the mass of the second body M
- 03:51
sub 2 divided by the square of the radius R whoo that made us a little dizzy
- 03:58
what do we do if we want to solve for R let's start playing with the equation [Person holding phone and formulas appear]
- 04:04
first off let's multiply both sides by r-squared algebra is always fair like
- 04:10
that both sides of an equation get equal treatment now our equation looks like
- 04:15
this r-squared times F sub G equals the product of G M sub 1 and M sub 2 now we
- 04:24
have to get r-squared all by itself so to isolate R squared we
- 04:29
divide both sides by F sub G like this leaving us with R squared equals G M sub
- 04:37
1 and M sub 2 over F sub G but wait we're trying to solve for R, not R [R and R squared waving]
- 04:44
squared so one more step if R squared equals let's just say everything on the
- 04:51
right we're tired of repeating all these letters sticking to the zen of algebra
- 04:55
we need to find the square root of each side to drill all the way down to R [A nail drilled through piece of dry wall]
- 04:59
since the square root of R squared is R that means R equals the square root of G
- 05:06
M sub 1 M sub 2 over F sub G boom! we've got R all by its little lonesome ready
- 05:15
to be solved now did you notice how we kind of breezed by G in this equation [Woman with arms wide in the breeze]
- 05:19
just dropped a gravitational constant in your lap and kept on moving well let's
- 05:25
take a closer look at what makes up that constant we're not going to find the
- 05:28
actual number for that constant we're just going to figure out what units it's
- 05:33
made of so we'll start back with F sub G equals G M sub 1 M sub 2 over R
- 05:40
squared multiply each side by R squared then divide each side by the masses M
- 05:46
sub 1 and M sub 2 so G equals F sub G times R squared over M sub 1 times M sub
- 05:56
but now we need to substitute units in
- 05:59
place of the variables that aren't G ...Force is measured in Newtons so F sub G
- 06:05
gets replaced with N... A radius is a length so that gets replaced with meters
- 06:10
and those meters are still squared then on the bottom we're dealing with masses
- 06:16
which means kilograms since it's kilos times kilos that means it's yup kilogram [Captain America appears below force equation]
- 06:23
squared so the units that make up the gravitational constant are newtons times
- 06:27
square meters / square kilograms not quite as simple as our old friend the [Meter standing on a chair]
- 06:33
meter now we can put everything together use some numbers and get some real
- 06:37
answers let's take one more look at the gravity equation we've been working with
- 06:42
yeah we're totally not tired of looking at it either but this time we're going
- 06:46
to use actual numbers and we're going to figure out the distance between the Sun [Distance between sun and earth appears]
- 06:51
and the earth and we're sure you remember we already arranged the
- 06:55
equation to solve for R... R equals the sub square root of G, M sub 1 and M sub
- 07:02
2 over F sub G time for those numbers we mentioned the gravitational constant is
- 07:08
6.67 times 10 to the negative 11th Newton's meters squared
- 07:13
over a kilogram squared see why we did that unit analysis just now Earth's mass [Earth appears in space]
- 07:19
is 5.98 to the 24th order of magnitude kilograms and the Sun
- 07:24
has a mass of 2 to the 30th order of magnitude kilograms
- 07:29
Wow the Sun is big and last but not least the force between the Sun and the
- 07:35
earth is 3.55 times 10 to the 22nd Newton when we put all those
- 07:40
into our equation for R it looks like this now we can start figuring this mess
- 07:46
out activate PEMDAS while we do have parentheses and exponents here they are [PEMDAS appears under an equation]
- 07:52
keeping the unit's clear and easy to understand so first off let's multiply
- 07:57
the numerator and while we're at it we can cancel out the kilograms since our
- 08:02
gravitational constant uses the inverse of kilograms squared
- 08:05
and our masses each used kilograms we're left with 7.98 times 10 to the 44th
- 08:12
Newton meters squared over 3.55 times 10 to the 22nd Newtons
- 08:18
oh yeah and we're still looking for the square root looks like it's time for
- 08:22
division and again we're able to cancel out a unit in this case Newton's after [Newtons cancelled out from formula]
- 08:28
we divide we find that R equals the square root of 2.25 times
- 08:33
10 to the 22nd meters squared doing our last bit of math tells us that the earth
- 08:39
is 1.5 times 10 to the 11th meters from the Sun or to put it a little less
- 08:46
scientifically really really far away so we've explored how to work with data [Independent and dependent variables in a table appear]
- 08:52
collecting it using the right significant figures for it making sure
- 08:56
we're using the right units for it all sorts of fun stuff but wouldn't it be
- 09:01
nice if we could actually look at our data really be able to see the pattern [Man examining data with magnifying glass]
- 09:06
of how changing an independent variable affects the dependent variable good news
- 09:11
graph paper exists graphing data lets us see how variables are related for now
- 09:17
we're going to just use a two-dimensional graph meaning we're [Graph appears]
- 09:21
going with the classic x and y coordinates when we're using a graph to
- 09:25
represent data from an experiment like the pendulum experiment we did the [Man and woman with swinging pendulum]
- 09:30
independent variable will be graphed horizontally on the x axis and if you
- 09:35
guessed that the dependent variable get graphed vertically on the y axis then
- 09:40
yeah it was the only option left but hey it was still a good guess let's do a
- 09:46
little refresher on some different kinds of mathematical relationships sometimes
- 09:51
we get lucky and have a nice pretty straight line something easy to deal [Straight line drawn through an axis]
- 09:55
with and in those cases we're going to pay attention to the line slope the
- 09:59
slope can be as steep or shallow it can be negative or positive and I could also
- 10:04
just be flat if going from left to right that line goes up then the slope is [Slope appears on graph]
- 10:09
positive if it goes down the slope is negative and if it's flat yep
- 10:14
that means the slope is zero and just in case someone wants to
- 10:18
get tricky on a test if you ever see a graph like this with a straight vertical [Graph with straight vertical lines]
- 10:23
line that means the slope is undefined which occurs when something is just
- 10:29
trying to mess with our heads or when we screwed up something in our math hey
- 10:33
everyone makes mistakes slope is rise over run
- 10:36
meaning rise meaning the y-axis and run meaning the x axis to put it more
- 10:42
mathematically the slope M equals the Delta of Y over the Delta of X in this
- 10:49
context Delta represents the change in value so the slope equals the change in
- 10:54
Y divided by the change in X if we have a steep slope of Mount Everest slope, that
- 11:00
means there's a big change in the value of y relative to the value of X and any
- 11:06
slope can be discovered using the linear equation which says that y equals M [Linear equation appears]
- 11:12
times X plus B and represents the slope and B represents the y-intercept which
- 11:18
is where the line crosses the y axis let's look at an example first what's
- 11:24
the slope of this line well we just need to look at any two points along the line
- 11:29
let's use the point at 3,10 and the point at 5,16 using our equation for slope we
- 11:36
find that M equals 16 minus 10 divided by F minus 3 [Equation for M appears]
- 11:41
making the slope equal to 3 so using that slope in the linear equation along
- 11:47
with the y-intercept value of 1 we can describe this graph using the linear
- 11:52
equation y equals 3x plus 1 of course not all lines are straight so that'd be
- 11:58
too easy we also have curved lines now there are all sorts of curved lines and [Person drawing curved lines on graph paper]
- 12:04
all sorts of nonlinear equations to go along with them but we're gonna focus on
- 12:09
three the first curved line we'll look at is the quadratic or squared line
- 12:15
it has a parabolic shape like this and the equation that describes it is y
- 12:21
equals AX squared plus BX plus C in this equation A, B and C are constants and you
- 12:29
may not need A or B if they're equal to 1 a quadratic line can have up to 2
- 12:36
roots and in this context a root is an intercept where the x value crosses the
- 12:42
x axis where the y equals 0 you can see these here at negative 1,0 and 2,0 the
- 12:51
next line up to bat is the rational line and the matching equation is y [Rational line appears]
- 12:56
equals A over X minus B you might also see this called an inverse function and
- 13:02
there's also a squared inverse that's y equals A over x squared minus B lastly
- 13:10
for this lesson is the radical line it's called a radical because the function is [Radical line appears on graph]
- 13:16
under a radical sign, not because it's really into surfing and because it uses
- 13:20
a square root it's also called a square root function and the equation is y
- 13:25
equals the square root of AX minus B and the line looks like a sideways parabola
- 13:31
see if we want to know the precise slope at a precise point on a curve we can get [Tangent curve appears]
- 13:38
it by finding the slope of a line that is tangent to the curve of that point it
- 13:43
would be great if we could see all of this graphing stuff in action if only we
- 13:47
had some data to put on a graph let's say like say we've done an experiment [Newton's cradle appears]
- 13:52
maybe with a pendulum and we'd written down a bunch of stuff measuring changes
- 13:58
to variables sound familiar? remember the independent variable is on
- 14:03
the x axis and the dependent variable is on the y axis [Table of dependent and independent variables]
- 14:08
so go ahead start to plot some lines on a graph see if you have a straight line
- 14:14
or a curve and if you have a flat line with a zero slope that means the
- 14:18
dependent variable isn't related to the independent one so changing the
- 14:22
independent variable doesn't affect the dependent so go ahead get plotting see [Person plots lines on a graph]
- 14:27
what direction the data points to have fun drawing your lines just don't get
- 14:32
too carried away if your graph ends up looking like a van Gogh painting maybe
- 14:35
save that one for art class instead of physics
Related Videos
When you're about to marry the love of your life, not many things could stop you. However, finding out that your future hubby is keeping his crazy...
Here at Shmoop, we work for kids, not just the bottom line. Founded by David Siminoff and his wife Ellen Siminoff, Shmoop was originally conceived...
ACT Math: Elementary Algebra Drill 4, Problem 5. What is the solution to the problem shown?
AP® English Literature and Composition Passage Drill 1, Problem 1. Which literary device is used in lines 31 to 37?
AP® English Literature and Composition Passage Drill 2, Problem 1. What claim does Bacon make that contradicts the maxim "Whatsoever is delig...