In our humble opinion, what you've learned so far is just the tip of the shmathematical iceberg when it comes to sequences and series. There is so much more out there. Some of these ideas will be essential to other courses. Other topics you may never be lucky enough to hear about. Imagine a world where you didn't know what a fractal was. Don't worry; we've got your back.
Fractals
Take fractals, for instance. If you're into art or graphic design even just a little bit, fractals are a sight for sore eyes. Go ahead, google it. The images are incredible.
What is a fractal? Good question. A fractal is a figure that has what's known as self-similarity. This is a very cool fractal:
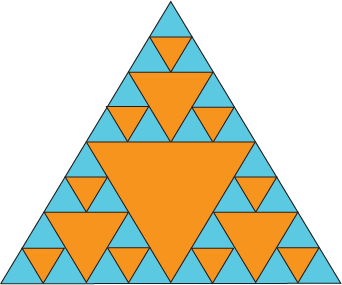
This fractal uses equilateral triangles. Notice the pattern. Each time another level of smaller triangles is inserted, more triangles appear. The number of triangles goes from 1 to 3 to 9 to 27. Holy moly. That looks awfully geometric to us. We're thinking a common ratio of 3 with a first term of 1.
Using a geometric sequence is just one way to design a fractal. There are plenty more. However, what's even cooler about fractals is they theoretically go on forever. Think about it like zooming in on an online map. The more you zoom, the more detail you find. Fractals can work in the same way. If we were to zoom into the fractal above, we could find another level with 81 small triangles, then 243, then 729, then 2187, then 6561. You get the idea.
Calculus II and Then Some
Calculus can sometimes be downright frightening, especially when it's followed by the Roman numeral II. However, after tying up a few loose ends from Calc I, Calc II courses typically jump into sequences and series. This is the real meat and potatoes of most Calc II courses.
This time around, the focus moves mainly to infinite series and proving whether they converge or diverge. Lucky for you, Shmoop has you ready to go with some fancy-pants Calc II vocab. By the way, if you need a refresher, the Glossary might be of assistance.
Where Calc II gets tricky is that there are more series types and tests to learn than just arithmetic and geometric. In fact, once you learn about these other series types, you'll start to create your own series from scratch. You'll even be able to take series and put x's into the rule, like this:

Believe it or not, if you start listing the terms of this series, you actually get an infinitely long polynomial.

What's amazing about this is that, for reasons you'll discover when Calc II finally rolls around, this series is actually a polynomial version of ex. And by a polynomial version, we mean that if you graphed this polynomial with all of its terms, it would be exactly the same as the graph of ex. Identical.
We find this to be fascinating. Especially when we can do the same thing for the graphs of sine and cosine. After all, how do you think your calculator evaluates sin(17) so quickly?