Sure, we could cut triangles in two until the cows come home, but there's an even simpler way to calculate the sides of a right triangle.
Mr. Pythagoras has once again come back to haunt us, doing what he's always done: finding distances. Just picture him, in all his ancient wisdom, enlightening us about the wonders of right triangles and how to find their side lengths.

"I do say, my dear chap. Methinks I've come up with a theorem: if a triangle is a right triangle, the sum of the square of its two legs equals the square of its hypotenuse. Indubitably so."

What Mr. Pythagoras is trying to say is that for any right triangle, a2 + b2 = c2, where a and b are the lengths of the two sides and c is the hypotenuse. (Don't forget, the hypotenuse is always the side opposite the right angle.) This is called the Pythagorean Theorem.
Mr. Pythagoras is probably telling us the truth. After all, it's hard to doubt a gentleman in a top hat. Just to be sure, though, we should double-check his work and prove his theorem using this triangle.

We can make two geometric means if we compare the hypotenuse to the long and the short legs of the big triangle.
1. Comparing the hypotenuse to the short leg gives us:

2. Comparing the hypotenuse to the long leg gives us:

If we cross-multiply each of them, we get a2 = cx and b2 = cy. Adding the two equations gives us the first half of Mr. Pythagoras's claim.
a2+ b2 = cx + cy
Almost done. If we factor the right side, we get:
a2+ b2 = c(x + y)
Looking at our original triangle, x + y = c. Plug in the last piece of the puzzle, and we have our final equation.
a2 + b2 = c2
Way to go. Now we can sleep soundly at night, knowing Mr. Pythagoras wasn't bluffing. Or, instead of sleeping, we can use his theorem to figure out some side lengths.
Sample Problem
What is the length of the hypotenuse of this triangle?

We just proved the Pythagorean Theorem. That's a good place to start.
a2 + b2 = c2
The only values we have are the lengths of the legs. We can substitute those in.
(3)2 + (4)2 = c2
Now we'll simplify as much as we can.
9 + 16 = c2
25 = c2
Taking the square root of both sides gives us the answer. Even though we know that square roots have two answers (a positive and a negative answer), lengths can't be negative. That's why we only care about the positive version.
c = 5
We've found the length of the hypotenuse. It's 5, by the way.
The hypotenuse is only one side, though. The other two sides of a right triangle are the legs. That means that there's a two out of three chance that we'll have to calculate the length of one of the legs and not just the hypotenuse.
Don't worry. It's not that much different.
Sample Problem
What is the length of the missing leg of this triangle?

Starting with the Pythagorean theorem again, we have a2 + b2 = c2. If we substitute in the lengths of the sides we already know, we'll get a2 + (1.5)2 = (3)2. Let's simplify what we can.
a2 + 2.25 = 9
a ≈ 2.6
We've gotten the hang of it by now. All right triangles follow the Pythagorean Theorem. What happens if we don't know whether or not it's a right triangle? If the three sides satisfy the Pythagorean theorem, then the triangle must be a right triangle. That's called the Converse Pythagorean Theorem.
Easy enough.
A square root sign turns most numbers from nice, whole numbers into long decimals. After Mr. Pythagoras came up with his theorem, he noticed how rare it was for a right triangle to have sides that were only whole numbers.

He decided set up a special resort called the Pythagorean Triple Club, complete with squash courts and a hot tub. A triangle that's a Pythagorean triple must have sides lengths of three whole numbers and satisfy his a2 + b2 = c2 equation. That means any right triangle with three whole-numbered sides has access to this Pythagorean Triple Club.
Example 1
Find the value of x. |
Example 2
Find the value of x.
|
Example 3
Is this a right triangle? |
Example 4
A triangle has three points at A (-10, -3), B (-5, -5), and C (-7, -10). Is ∆ABC a right triangle? |
Exercise 1
Find the value of x.

Exercise 2
Find the value of x.

Exercise 3
Find the value of x.

Exercise 4
A triangle has side lengths of  ,
,  , and
, and  . Is the triangle a right triangle?
. Is the triangle a right triangle?
Exercise 5
Determine whether ∆XYZ is a right triangle and/or a Pythagorean triple if its vertices are at the points X (1, 1), Y (1, 7), and Z (9, 1).
Exercise 6
If the distance between A and B is 26, what is the value of y?

Exercise 7
Find the value of x.




 , where d is the distance and x and y are the coordinates of points 1 and 2. We'll need to do this three times, so let's get to it.
, where d is the distance and x and y are the coordinates of points 1 and 2. We'll need to do this three times, so let's get to it.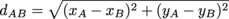

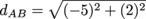
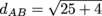
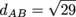
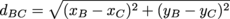
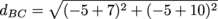

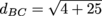
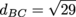
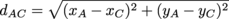
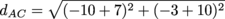
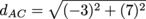
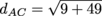
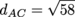
 ,
,  , and
, and  . Those are the lengths of the triangle's sides. They don't tell us much alone, but if these numbers satisfy the Pythagorean Theorem, that means the triangle is right.
. Those are the lengths of the triangle's sides. They don't tell us much alone, but if these numbers satisfy the Pythagorean Theorem, that means the triangle is right. 
