Solving Systems of Linear Equations by Graphing Examples

Solve the following system of equations:

The first equation is in standard form, which we can graph by finding the intercepts:

The second equation is in point-slope form. We can graph this equation by rewriting it in slope-intercept form to get

and then graphing:

These lines are parallel, which means they will never intersect. They'll always be able to glance over and wave at the other, but they'll never be able to shake hands or slow dance. A pity, because they can tear it up on the dance floor.

There's no solution to this system of linear equations.

Solve the following system of equations:

We graph the first equation using the slope and intercept, and the second equation using the intercepts: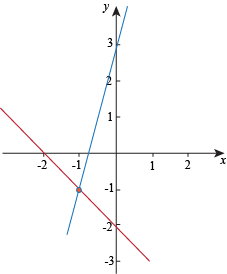

These lines appear to hit right at (-1, -1). Let's check and make sure they work. If they don't, we may need to have our negatives developed. #filmcamerajoke

The first equation was y = 4x + 3. When x = -1 and y = -1, the left-hand side of this equation is -1 and the right-hand side is

4(-1) + 3 = -1.

This point works in the first equation.

The second equation was -3x – 3y = 6. When x = -1 and y = -1 the left-hand side of this equation is

(-3)(-1) – 3(-1) = 3 + 3 = 6,

which is the same as the right-hand side of the equation.

Since the point (-1, -1) does indeed satisfy both equations, we can safely say that (-1, -1) is a solution to the system of equations. We feel even safer saying it now that that guy with the scythe has left the room. What was he even doing here?